Mental Multiplication using Vedic Math - Part 2
Use Indian Vedic Techniques to excel in Math
Introduction
This is the part 2 post of the series on “Mental Multiplication using Vedic Math”. Here we will find out on “How to Multiply 2 numbers that are greater than a common 10x “. Example: 104 * 109
If you need to refer part 1 post, pls click here
We will still use the same rule “Nikhilam Navatashcaramam Dashatah” meaning “All from 9 and the last from 10”.
Can you do this below multiplication mentally in few seconds ?
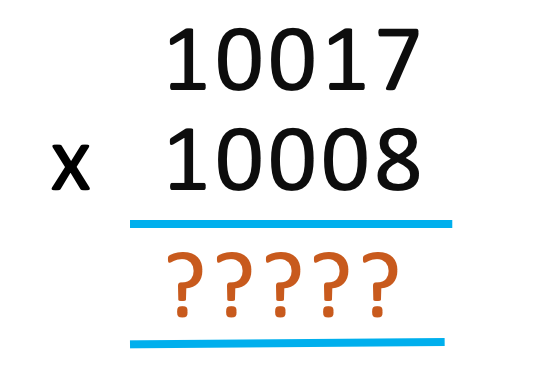
If this interests you, then this series of post is for you. Read on to find out how we can do this in few seconds.
Power of 10 Base
Just a recap - For Nikhilam multiplication, we need to associate numbers close to their corresponding 10x base. If you need to under more on how to identify the base, pls read the previous post here
Examples of Nearest Base as 10x:
| Number (N) | Nearest 10x | Value of 10x | Difference (N - 10x) |
|---|---|---|---|
| 8 | 101 | 10 | -2 (= 8-10) |
| 13 | 101 | 10 | +3 (= 13-10) |
| 89 | 102 | 100 | -11 (= 89-100) |
| 105 | 102 | 100 | +5 (= 105-100) |
| 225 | 102 | 100 | +125 (= 225-100) |
| 775 | 103 | 1000 | -225 (= 775-1000) |
| 1200 | 103 | 1000 | +200 (= 1200-1000) |
and so on…
So in the Nikhilam Multiplication that we will see soon, we would be referring to these actual numbers and as well as the difference that we calculated from their correponding bases for calculations. Also note the sign for the differences as that is going to play a very important role in multiplication!
What is Nikhilam Sutra ?
Pls read the previous post here to understand more on this.
How Nikhilam Method Works?
Let us see, “How to Multiply 2 numbers that are greater than a common 10x”. Example: Find the value of 104 x 109 ?
Nikhilam Method Working:
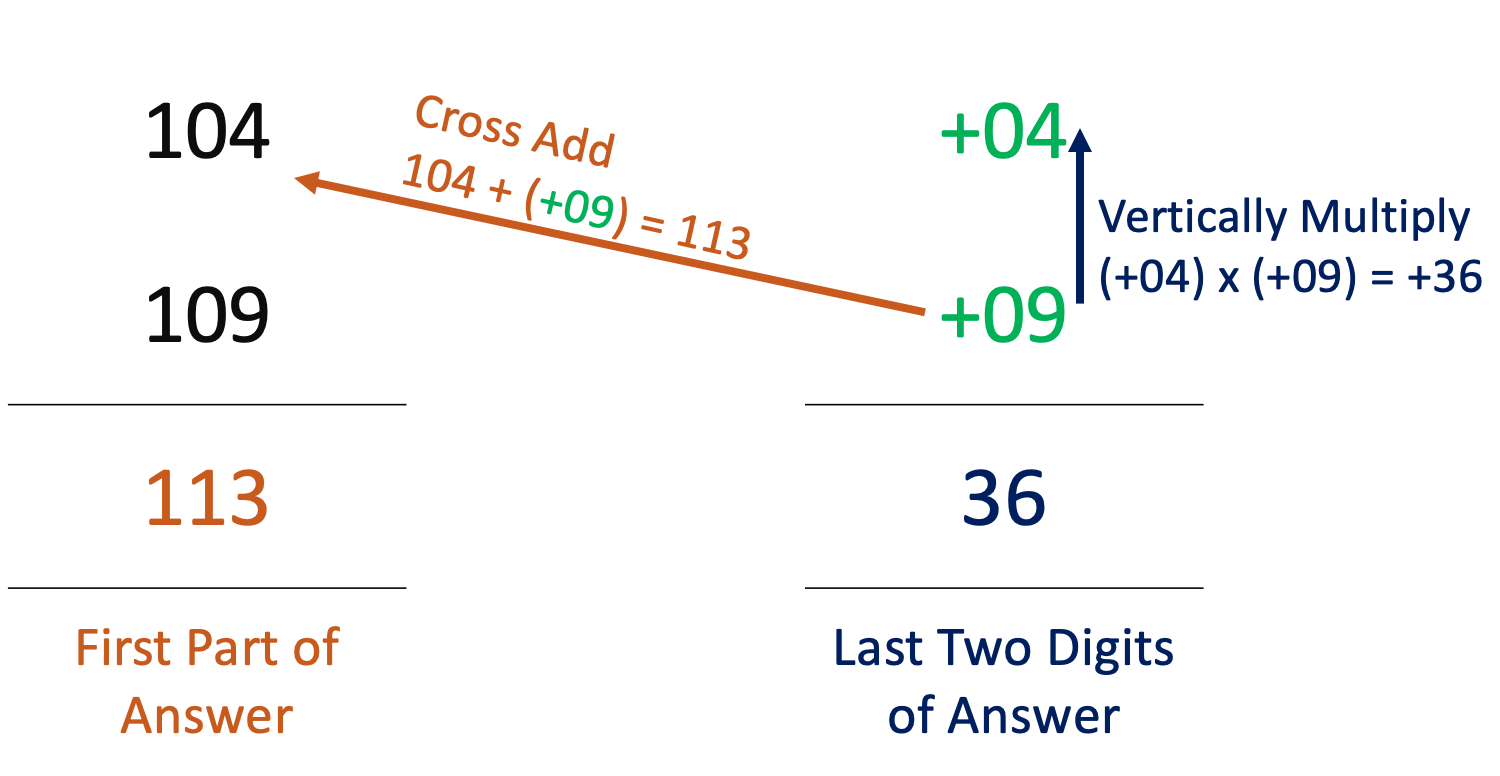
So, 104 x 109 = 11336
Steps to perform the Nikhilam Method:
- Lets first get their corresponding 10x & the difference from 10x.
- Write the numbers that you want to multiply one below the other
- Write the difference between the number and the closest base 10s number on the right side of the number.
- Since the numbers are greater than the base, the difference is written as positive numbers
- Now multiply the differences. Both numbers are positive. Hence the multiplication will be a positive number. Based on the base that you have chosen (here we have chosen 100), product of these two differences should be written in as many digits as zeros' in the 10x. Like here product of the difference is 04 * 09 = 36. Since base 100 has 2 zeroes, the answer should be noted in two digits. 36 is a 2 digit number so no issues otherwise we would have to add a leading 0 zeroes to fill that gap. Write the product down.
- Both the numbers on left side are greater than the base. Hence you can take any of the difference from right side and add it crosswise from the other number on the left. That is either 104 + 09 or 109 + 04. Both will yield 113. Write this number down.
- There you go the answer is 11336.
| Actual Numbers to Multiply | Corresponding Difference from 10x |
|---|---|
| 104 | +04 |
| 109 | +09 |
Algebric Proof for Nikhilam Method
Are we sure that this method will work correctly? Or is it just a mere coincidence?
Though the steps might seem so absurdly simple, internally Vedic Math follows every single mathematical principle and there is absolutely no deviation in that.
Like previous post, lets see the Algebric Proof for this as well.
Since the numbers to be multiplied can be represented as (10x + a) lets define the numbers A & B as follows.
Let
A = (10x + a)
B = (10x + b)
So A x B = (10x + a)(10x + b)
= (10x)2 + 10xa +10xb + ab
= (10x)(10x + a) + (10x)b + ab
= (10x)(A) + (10x)b + ab
= (10x)(A + b) + ab
This is what we are doing in Nikhilam.
Cross add for first portion of the answer = A + b
Vertically multiply for the last portion of the answer = ab
Isn’t it cool!
Now you will be able to do this math right in your head and you wouldn’t need a calculator or a piece of paper. This can be extended to any higher order base 10s as well. And it will just work very fine.
See below for additional examples:
1
2
3
4
5
1502 + 502
x 1002 + 002
-----------------
1504 1004
------------------
Important: We already said that the right side should have digits equivalent to as many zeros in your base 10x. But here we have 4 digits on the right side whereas the base 10x (1000) has only 3 zeroes. Hence we carry forward the excess digit (1 in this case) to the left side number (1504).
So answer is 1505004.
1
2
3
4
5
1001 + 001
x 1008 + 008
-----------------
1009 008
------------------
Important: Since 1000 is the (10x) we have chosen, the mulitplication of the differences should be represented in 3 digits (as many zeros in (10x) chosen). Hence leading zeroes for 8!
So the answer is 1009008.
Conclusion
So lets conclude the post by finding the answer for the question.
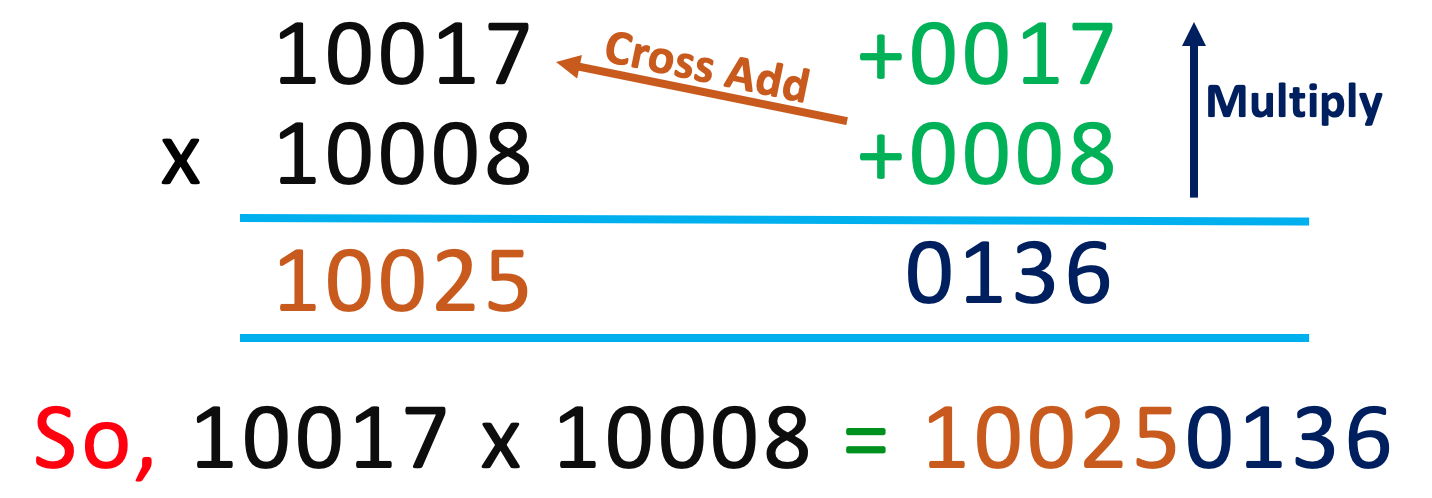
If you were to do this in regular method, it would have been very very laborious. See below.

We will look into the other variations in the subsequent posts. Until then, happy mental math!

 Never miss a story from us, subscribe to our newsletter
Never miss a story from us, subscribe to our newsletter